Today, Tiptop Audio announced the official release of the new Tiptop/Buchla 266t Source of Uncertainty—a Eurorack module inspired by one of the most peculiar, iconic, and downright significant pieces of modular synthesizer technology of all time.

Why Would You Want Randomness In Your Music?

For some background on the topic of uncertainty in music, I'd strongly recommend checking out our Learning Synthesis series, where Naomi Mitchell of omiindustriies gives us insight into the historical relationship between randomness and electronic music, as well as the early history of randomness in Don Buchla and Serge Tcherepnin's instruments. These are an excellent starting point for our present discussion—and we'll certainly re-tread some ground that Naomi already covered—but, let's proceed here by giving a recap of some basic ideas.

Why do we care about randomness in music? For some people, randomness is the opposite of what they want in their music: the Romantic vision of the artist carries with it a lot of baggage, including a common notion that an artist with a fully-formed, complete, meticulous vision has somehow better odds of greatness—or better opportunity to be extensively appreciated—than an artist who doesn't provide equal consideration for every single detail of the things that they create. It's why people love an "auteur": when it's clear that so many decisions were made by a single person when creating something, it's easy (and even fun!) to scrutinize every microscopic detail in order to glean greater meaning/greater understanding of the work as a whole.

In the mid 20th century, it became increasingly common for experimental musicians to, well, go a different direction. As time went on, and as science became more concerned with the potential applications of cybernetic processes and computation, it became fairly commonplace for musical composers to employ procedural systems in the creation of their music. Serialism, set theory, minimalism, all of it—they are ways of establishing systems by which the details of a piece of music can be extrapolated. It was no longer about specifying every single note that an instrumentalist should play...instead, it was common for composers to relegate decisions like that to processes that would determine the lower-level details. From there, of course, embellishments and finer details about expression, orchestration, and any other aspect of composition could be applied. Being a composer was, for many, a type of algorithmic system design. I'm not going to go deep into the history of musical modernism, or to the divide between modernism and postmodernism in so-called "art music," but if you want some interesting examples, you could look to music by Milton Babbitt and John Cage (each pictured above), for instance, for quite distinct stylistic/conceptual approaches to this type of composition-by-algorithm.

Of course, as academic composers created these new means of musical organization, experimentation was also taking place with respect to the use of electronics in music. It was already relatively commonplace by the 1960s for cutting-edge composers to experiment with electronic media, using tape studios, test tones generators, equalizers, etc. Many studios even had specialized instruments purpose-built for the creation of electronic music: I'm thinking of the RCA MkII Synthesizer at the Columbia-Princeton Electronic Music Center (created by Harry Olson and Herbert Belar), the studios of McGill University and the University of Toronto (with many devices designed by Hugh le Caine), etc.

×

But when the original modular synthesizers came along, they provided unique promise: an unprecedented degree of real-time control, allowing not only experimentation with sound, but also experimentation with musical structure. Early modular synthesizers by Don Buchla and Bob Moog—each initially embraced by experimental composers and academic institutions—privileged non-traditional approaches to musical structure. They each featured sequencers, touch-sensitive controllers, and...random voltage generators. This meant that composers who were interested in procedural composition had a broad spectrum of possibilities at their fingertips for automatically creating and auditioning musical ideas, with the absolutely deterministic potential of a sequencer, for instance, on one end of the spectrum and complete randomness on the other. It would seem that, somewhere between these two extremes, quite a lot of exciting musical territory was waiting to be discovered. The ability to absolutely specify a behavior on one hand, and the ability to specify scenarios in which you wanted something uncertain to happen—and the ability to apply that uncertainty to any aspect of sound, be it pitch, loudness, timbre, or even aspects of the musical structure itself...well, let's just say it meant that there was a lot to discover. (Note—you can read more about these ideas in our article What is a Modular Synthesizer?)

So again...why would you want to use randomness in your music? Wouldn't a composer want absolute control over every aspect of their creations? Well, no, not necessarily—in fact, it's easy enough to re-frame that thought by suggesting that the element of uncertainty is itself a compositional decision, one that carries with it a number of other decisions. What aspect of the sound is the uncertainty applied to (pitch, timbre, loudness, rhythm, or other details of the musical structure itself, perhaps)? What degree of uncertainty is applied (is all over the map, or constrained to a small amount of deviation)? When is uncertainty applied (is it constantly changing, or does it only change in coincidence with another significant action)?

Once you realize that the use of randomness in music does still require quite a lot of careful musical decisions, it's suddenly easier to open ourselves up to considering the quite interesting potential it offers. Randomness can be a way of instilling an element of surprise, both to a listener and even to a performer or the composer themselves. Randomness can be a way of discovering inspiring new sounds. Randomness can be a way of unearthing combinations of parameters you might never have otherwise considered. Randomness can be a way of granting an instrument some degree of autonomy. In fact, randomness can be a way of reframing your entire relationship with your instrument—turning into something that is always transforming, both in ways that you can roughly predict and in ways that you could never foresee. To me, that's all quite exciting, and quite inspiring.

Before the Buchla 266: Another Source of Uncertainty

Don Buchla extensively embraced the potential of randomness, perhaps more so than any other instrument maker in the 1960s and 1970s...perhaps more so than any other instrument maker to this very day. In fact, to many, uncertainty is key not only to Buchla's instruments themselves, but to his entire legacy: the sense of mystery surrounding his life, his musical intentions, and the devices he created is a significant part of their appeal. In fact, oftentimes, myth and mystique is more interesting than truth. Often attributed to educator and Buchla Associate Allen Strange, it is presumed that the phrase "source of uncertainty" itself includes a subtle nod to this aspect of Buchla's work, and always has.

×

I'll add a quick note here: if you're interested in my thoughts about the significance and impact of Don Buchla's work—as well as what made him so distinct from other instrument makers—I'd like to suggest that you check out a few more articles that we've published. Our Tiptop Audio + Buchla 200 Series Overview offers a high-level overview of what makes Buchla's work so special; and our two-article series East Coast Synthesis vs. West Coast Synthesis + What is West Coast Synthesis...Really? attempt to provide a deeper look at the historical context surrounding his work and its influence on modern designs. They all make for a fun read.

When most refer to the Source of Uncertainty, they're referring to the Buchla Model 266—the subject of Tiptop's reimagined Eurorack module, which we'll discuss shortly. However, there were random sources in Buchla's lineup prior to the 266, and there was even a prior Source of Uncertainty. In the 1960s 100 Series, the 165 Dual Random Voltage Source was the primary means of generating random control voltages—it was quite a simple module, with two pulse inputs, each with their own set of CV outputs. When the module received a pulse, the voltage at the outputs for each respective section would update to some new random voltage level. This enabled randomization of pitch, timbre, timing intervals, you name it. However, it wasn't so different from, say, patching noise into a sample-and-hold circuit. So when Buchla moved on to designing his next instrument, in which basically everything received a much finer degree of control, he clearly asked the question: how could he make a module that enabled greater control over randomness?

×

Thus, with the introduction of the 200 Series in ~1970–1971, he introduced the 265 Source of Uncertainty (see the second image in the carousel above). The 265 offers considerably more control than the 165, organized into three sections: White Noise Outputs, Random Voltage Outputs, and Stored Random Voltage Outputs. The Noise section offers three distinct sonic flavors of noise: low (emphasizing low frequencies, a la pink noise), high (emphasizing higher frequencies), and an unlabeled more neutral noise setting. The "Random Voltage" setting provided two distinct fluctuating random voltage sources—continuously fluctuating, smooth random signals with variable control over their probable rate of change. Heck, they even offered voltage control of their rate of change, making it possible to use control sources to vary the speed of the fluctuation.

The Stored Random Voltage section was much like the 165: two pulse inputs, each with their own dedicated output, producing a new stepped random voltage with each incoming pulse. However, it had a key difference: each output had a dedicated Correlation control. This control, when set to minimum, made it such that the output was truly random for each new pulse. However, as you turned the Correlation control up, new random voltages would get closer and closer to the most recent random voltage...basically acting like a "deviation" control. You could make it such that, at higher settings, new voltages would deviate only slightly from the prior voltages, allowing changes to unfold over longer periods of time, or simply allowing for more subtle changes from pulse to pulse.

Random voltage section detail from a modern clone/work-alike of a Buchla 212 Dodecamodule

×

There were other Buchla modules with random voltage generators built in. The 212 Dodecamodule included a simplified, 265-like set of possibilities in its design, and the later 208 Stored Program Sound Source (the Music Easel sound module) included a quad stepped random voltage generator.

Perhaps here is a good time to mention that there's quite a significant shift in approach and functional density between earlier and later designs in the Buchla 200 Series—which was developed throughout the 1970s. Many designs from the early 1970s can seem quite crude by comparison to their later counterparts, many of which are considerably more sophisticated...and considerably more peculiar. And in fact, when most people talk about the 200 Series, what they're really referring to are mostly designs from the mid-late 1970s. This is where we find the 266 Source of Uncertainty.

Let's Get Stochastic: the 266 Source of Uncertainty

Tiptop's reimagining of the 266t retains much of the functionality of a real Buchla 266, adapted for use in Eurorack systems. It uses Eurorack signal levels, 3.5mm patch cables, and will fit nicely in your Eurorack case and plug right in to your Eurorack power supply. So, it's at this point the closest thing you'll be able to find in Eurorack format to approximate the behavior of the 266. Details about layout and subsequent graphic design changes aside, this is electronically the equivalent of what a 266 should do. So, let's talk about how it works! (Pictured above: a modern replica of the Buchla 266 produced by company Buchla USA; followed by the Tiptop/Buchla 266t.)

The top of the 266 is much like the 265 we discussed above: a noise source with three distinct colors of noise, now labeled as "-3dB/octave" (pink-ish noise), "+3dB/octave" (blue-ish noise), and "flat"—a perceptually balanced noise with equal energy distribution across the audible frequency range (slightly gentler than typically harsh "white" noise). From there, we encounter another familiar section: Fluctuating Random Voltages, with two continuously fluctuating smooth random voltage sources, each with dedicated controls for probable rate of change and corresponding CV inputs. From there, though, things get more complex.

×

The 266 has three sections dedicated specifically to producing stepped random voltages: Quantized Random Voltages, Stored Random Voltages, and a Sample & Hold section. First, let's talk about the Quantized Random Voltages section...something quite distinct and unique to the 266. This section allows for control of the number of states available to two outputs, respectively labeled n+1 states and 2^n states. This nomenclature alone is enough to make some people recoil, so what does all that actually mean? Well, think of it this way: with a typical noise-into-sample-and-hold patch, you have an infinite selection of potential outcomes. It's truly random, in that any state within the total output voltage range is possible. The Quantized Random Voltage section on the 266 makes it such that you have control over the number of potential outcomes via the "quantization" knob, which has a range of 1–6. The current setting of that knob can be conceptualized as the n value, and it affects both the n+1 and 2^n outputs. With quantization set to 2, for instance, the n+1 output will choose between three potential voltages (2+1)—with quantization at 6, it'll choose between seven voltages (6+1). You can apply the same logic to the 2^n output: with quantization at 4, for instance, 2^n chooses between 16 values. Whoa.

There are interesting implications to consider about the way this section is electronically implemented (some of which is discussed in this article). Both outputs update simultaneously when a pulse is sent to the QRV pulse input, and the n value may be voltage-controlled, allowing for automated control of the number of potential output values. In fact, interestingly, the n value can be updated continuously without needing a pulse input—so you can do quite bizarre things sending a CV signal into the quantization CV input, using the QRV section like a peculiar and quite screwed-up, nonlinear voltage quantizer. There are other, subtler quirks: the 2^n output has uniform voltage distribution, but the n+1 output tends to favor voltages in the middle of its output range. Also, running at higher rates, you'll realize that the outputs aren't really random, per se—they actually repeat after a certain point. All in all, there's a ton to be explored in this section alone—random coin tosses, peculiar quantization, high-speed irregular sequencing...it's all up to your imagination.

×

Next, the Stored Random Voltage section is another source of stepped random voltages—again, with a single pulse input that updates two outputs. One output offers more-or-less uniform voltage distribution, producing a new random value with each pulse...but the other output also features a probability distribution control, which allows you to continuously vary the likelihood that the output voltage will be low, high, or anywhere in-between. Like the quantization control in the QRV section, the distribution control can be continuously controlled between incoming pulses...so you can send a CV into it even without applying a pulse and get a peculiar, mutated version at the output. Each new pulse will apply a different transfer function to the output, making for a highly irregular means of "voltage processing" in which you can easily access a "randomized" version of the input signal. It's a weird, sort of backwards way of thinking about what that section is for, but hey, that seems like fair game to me.

In a module like the 266, even the Sample and Hold section has to be more than a simple sample and hold. Looking closely, you'll notice that the S&H section has a single CV input, a single pulse input, two pulse outputs, and three CV outputs...whereas a simple sample and hold would have only a pulse input, CV input, and CV output, right? Well, in actuality, the 266's S&H section is three sample and holds and a two-stage pulse sequencer/counter. Let's examine how it works more closely.

×

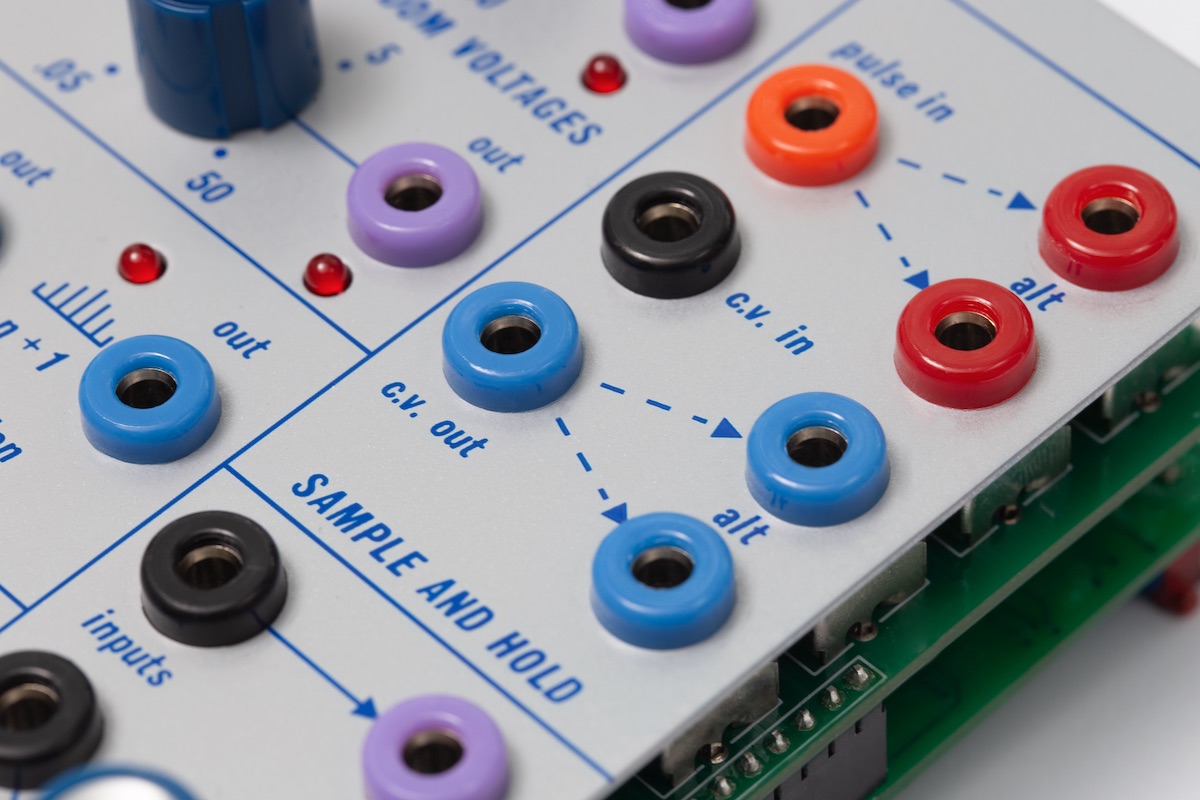
The bottom three jacks on the original and left three jacks on the Tiptop/Buchla module are a simple sample & hold—you send a pulse in and a CV input, and you get a sampled version of the CV input at the CV output with each new pulse. You'll notice the pulse input is visually connected to two pulse outputs labeled alt. These pulse outputs alternate with each incoming pulse—first one goes high, then the next, the the first again, and so on. These pulses are in turn used to trigger two additional alternating sample & hold outputs, which as a result update staggered from one another with every other input pulse.

This might seem kind of bizarre, but it's actually quite interesting...and if you're familiar with early Buchla 200 systems (read as: if you're as dorky as me), then you might note a similarity to the 264 Quad Sample and Hold / Polyphonic Adaptor. In fact, you can use this section as a polyphonic adaptor by which a monophonic controller (like a sequencer or monophonic CV keyboard) can be used to address two completely independent voices in round-robin fashion. You send your control source's pitch CV into the CV input and a pulse into the pulse input, and then connect the alternating outputs to two oscillators' pitch CV inputs and envelopes' pulse inputs et voila! Suddenly your monophonic controller is polyphonic. (You can read more about this approach in this article about voice allocation in vintage synthesizers.) Of course, there's much more you can do with this section...that's but one simple, practical example.

The 266 is rounded out by an integrator: a slew limiter with voltage control of the lag time/integration rate. This combined with the stepped random voltage sections can be used for a host of effects from portamento to programmatically-determined voltage transition times.

Seem Complex? Well...It Is!

Okay, so that's all been a significant mouthful—this module is dense, and it makes a lot of things possible that other instruments simply can't approach. You might be wondering...how the heck could I possibly use all of this for music?? It seems like an awful lot of math and technical concepts for something that's supposed to be about art and expression, right?

Well, yes! But remember—the original purpose of electronic instruments was about finding new ways of exploring expression. They were about uncovering what all new possibilities could emerge through tight interaction between humans and machines. Buchla's instruments, more so than most, consistently retained this perspective as they were developed. So, while many other makers in the 1970s had pivoted toward producing more portable, affordable, easily comprehensible designs, Buchla was instead trudging forth into the unknown: constantly asking questions about what might be possible rather than conforming to any pre-conceived notion about what music was or how it should work. As such, you can look at his instruments as a constantly evolving experiment in human-machine interaction. They had experimental functions, experimental interfaces, and experimental means of control. If it's not obvious to you how something might be used musically, well, that's more or less by design: these instruments were created for the purpose of confronting you with possibilities you might not have considered...for the purpose of putting you in a place of uncertainty.

When you're faced with more musical options than were previously available to you, what do you do? If you're presented with a tool that offers a new way of solving a familiar problem, will your approach to the problem change? If suddenly everything is possible, what will you choose to focus on? These are the types of questions that come along with using Buchla's more sophisticated instruments—and if you approach them sincerely, the best possible outcome is that you'll learn quite a lot about your own artistic values and musical vision.

As you're hopefully starting to see, uncertainty isn't necessarily a bad thing. Uncertainty means new possibilities—new areas to explore—heck, it might even mean new ways to feel, and new ways to communicate the way that you're feeling. I'll ask again: if every possible sonic option and every possible mode of interaction from strict control to absolute uncertainty is available to you, what will you choose to do? What will you choose to focus on? That thing might just be the truest form of your personal creative voice/vision, and one of the most magical aspects of these instruments is that they were designed in such a way that they can't help but help you discover it.